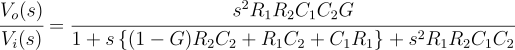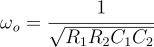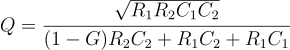Sallen-Key filters
What are Sallen-Key filters?¶
The Sallen–Key topology is used to implement second-order active filters that easily enhance the Q of the filter using controlled positive feedback. The topology is more simple in comparison to other active filter topologies. In 1955, R. P. Sallen and E. L. Key described these filter circuits, and hence they are generally known as Sallen-Key filters.
While Sallen-Key might be more beneficial in terms of component count and ease of use, we can achieve better distortion and stability in Multiple-Feedback Filter implementation.
Second order passive low-pass filter¶
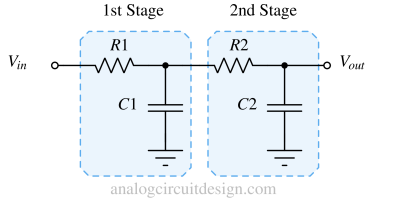
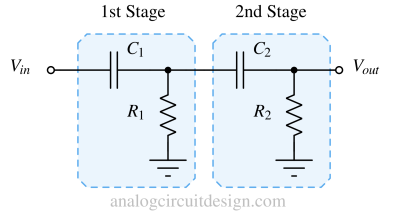
Figure 1 shows a RC-RC network that forms a second-order low-pass filter. With R1=R2=R and C1=C2=C the quality factor Q becomes 1/3. Q approaches the maximum value of 1/2 if the impedance of the second RC stage is made much larger than the first. Most filters require Q larger than 1/2.
The transfer function of a passive second-order low-pass filter is :
$$\cfrac{V_{out}}{V_{in}}=\cfrac{1}{s^2R^2C^2+3sRC+1}$$
$$Q=\cfrac{1}{3}$$
$$\omega{}_o=\cfrac{1}{RC}$$
Sallen-Key low pass filter circuit¶
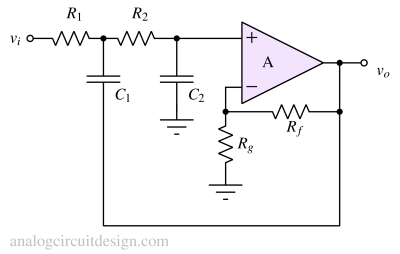
Larger Qs are attainable by using a positive feedback. If the positive feedback is controlled, and localized to the cut-off frequency of the filter, almost any Q can be realized. Obtaining higher Q is limited mainly by the component tolerances and opamps gain-bandwidth product. The filter cutoff frequency should be much less than opamps's gain-bandwidth product. Figure 2 shows an opamp in non-inverting gain used in this manner. Capacitor C1, which is not connected to the ground, provides a positive feedback path.
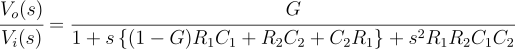
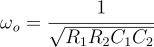
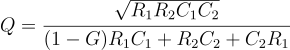
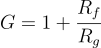 If R1=R2=R and C1=C2=C, the Q factor is :
If R1=R2=R and C1=C2=C, the Q factor is : 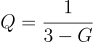
From the above equation, it can be concluded that Q > 1/2 can be achieved if Gain (G) > 1. This achievable Q is higher than any passive filter.
Also, keeping G greater than or equal to 3 will make the system unstable. This is because it will cause at least one pole to appear on the right-hand side of s-plane. This results in either perpetual oscillation or exponential growth in the output amplitude.
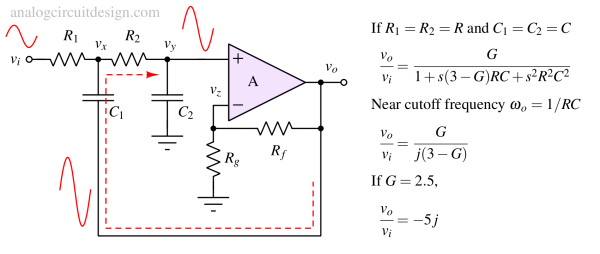
Intuitively, we can understand the operation in the following steps:
- At low frequencies, where C1 and C2 appear as open circuits, the system behaves as an opamp in a non-inverting configuration providing a gain of G (=1+Rf/Rg).
- At high frequencies, where C1 and C2 appear as short circuits, the signal is attenuated at the amplifier’s input, the amplifier amplifies this input to its output, and the signal does not appear at Vo.
- Near the cut-off frequency, if gain G is set close to but less than 3 (e.g., 2.5), Vo becomes very high which is basically feeding current through C1 from Vo to vx. This increases the input at the non-inverting pin (vy) resulting in high Q of this filter.
Second order passive high-pass filter¶
Figure 4 shows a two-stage RC network that forms a second-order high-pass filter. For simplicity, if R1=R2=R and C1=C2=C. This results in Q=1/3. This low Q results in attenuation in the passband and not enough attenuation in the stop band. A higher Q is desired. A higher Q is achievable using an opamp and some positive feedback. This is similar to how the Sallen-Key low-pass filter is made.
The transfer function of a passive second-order high-pass filter is :
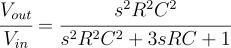
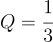
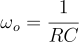
Sallen-Key high pass filter circuit¶
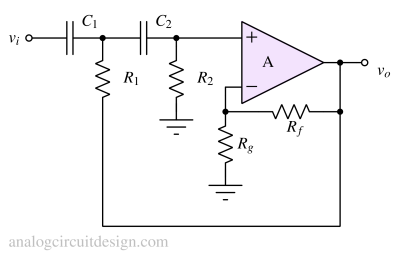
Figure 5 mentions a Sallen-Key high pass filter. Instead of R1 connected to the ground, it is connected to the output of the amplifier to provide positive feedback. Intuitively, we can understand the operation in the following steps:
- At low frequencies, where C1 and C2 appear as open circuits, R2 is pulling down the non-inverting node to the ground. This means no signal is getting amplified.
- At high frequencies, where C1 and C2 appear as short circuits, vi completely appears at the non-inverting pin of the opamp because the impedance of C2 is lower than R2.
- Near the cut-off frequency, similar to the Sallen-Key low pass filter, a current is injected through R1 into C2 from the Vo node. This leads to higher signal strength at the non-inverting pin which results in higher Q.